Worked example: Derivative of Ã(3x_-x) using the chain rule | AP Calculus AB | Khan Academy

Khan Academy
28 Jan 201305:31
EducationalLearning
32 Likes 10 Comments

TLDRThis video script introduces the concept of the chain rule in calculus, explaining its abstract formula and demonstrating its application through a concrete example. The chain rule is used to find the derivative of a composite function, expressed as f(g(x)). The video walks through the process of defining functions f and g, applying the chain rule to find f'(g(x)), and then differentiating the inner function g(x). The example involves differentiating the square root of (3x^2 - x), and the video clearly illustrates each step, making the concept accessible and engaging for learners.

Takeaways
  • 📌 The video begins with an introduction to the formula for the chain rule in calculus.
  • 🔢 The chain rule is used to find the derivative of a composite function, expressed as f(g(x)).
  • 🎨 The presenter emphasizes the importance of accurate use of colors to represent functions and their derivatives.
  • 🚀 The goal is to calculate the derivative of f(g(x)) with respect to x, which the chain rule states is f'(g(x)) * g'(x).
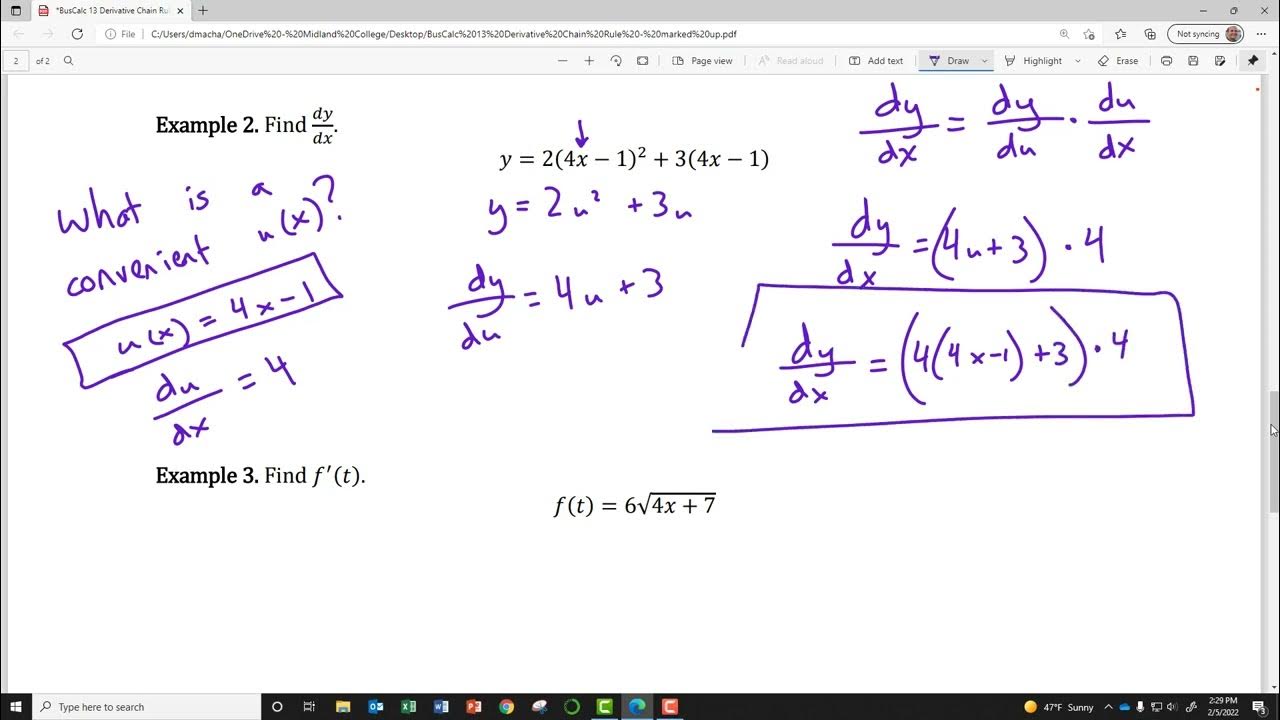
  • 🌟 An example is provided to illustrate the application of the chain rule: finding the derivative of the square root of (3x^2 - x).
  • 📌 Functions f(x) and g(x) are defined to facilitate the application of the chain rule to the given example.
  • 🔍 The derivative of f(x) is found using the power rule, resulting in f'(x) = 1/(2*x^(1/2)) or f'(x) = x^(-1/2).
  • 📈 The derivative of g(x) is calculated as g'(x) = 6x - 1, using the power rule for each term in g(x).
  • 🧩 The final derivative is obtained by multiplying f'(g(x)) * g'(x), which is 1/2 * (3x^2 - x)^(-1/2) * (6x - 1).
  • 📝 The process demonstrates the step-by-step application of the chain rule and the power rule in calculus.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is the chain rule in calculus and how to apply it to the derivatives of composite functions.

  • How is the chain rule expressed mathematically?

    -The chain rule is expressed as the derivative of the outer function with respect to the inner function, multiplied by the derivative of the inner function with respect to the variable x.

  • What is the notation used to represent a function composed of two functions?

    -The notation used to represent a function composed of two functions is f(g(x)).

  • What is the example function given in the video?

    -The example function given in the video is the square root of (3x squared minus x).

  • How are the functions f(x) and g(x) defined in the example?

    -In the example, f(x) is defined as the square root of x, and g(x) is defined as 3x squared minus x.

  • What is the derivative of f(x) with respect to x?

    -The derivative of f(x) with respect to x is (1/2)x to the power of negative 1/2, which is derived using the power rule.

  • How is the chain rule applied to the example function?

    -The chain rule is applied by first finding the derivative of the outer function f with respect to the inner function g(x), and then multiplying it by the derivative of g(x) with respect to x.

  • What is the derivative of g(x) with respect to x?

    -The derivative of g(x) with respect to x is 6x - 1, found by applying the power rule to each term in g(x).

  • What is the final expression for the derivative of the example function using the chain rule?

    -The final expression for the derivative is (1/2)(g(x)) to the power of negative 1/2, times (3x squared - x), times (6x - 1).

  • How does the chain rule help in understanding the behavior of composite functions?

    -The chain rule helps in understanding the behavior of composite functions by allowing us to find the derivative of a complex function by breaking it down into simpler functions and analyzing their individual and combined effects on the derivative.

  • What is the significance of the chain rule in calculus?

    -The chain rule is significant in calculus as it is a fundamental tool for differentiating composite functions, which are common in many real-world problems and advanced mathematical concepts.

Outlines
00:00
📚 Introduction to the Chain Rule and its Application

This paragraph introduces the concept of the chain rule in calculus, emphasizing its importance in determining the derivative of composite functions. The speaker begins by explaining the abstract formula for the chain rule and then illustrates its application through a concrete example. The example involves finding the derivative of the square root of (3x squared - x) and defining functions f(x) and g(x) to represent this expression. The process of applying the chain rule is detailed, showing how to calculate the derivative of the outer function with respect to the inner function, and then the derivative of the inner function with respect to x. The explanation is enriched with the use of color to keep track of variables and maintain clarity throughout the process.

05:04
🔢 Conclusion and Review of the Chain Rule Application

In this paragraph, the speaker concludes the application of the chain rule from the previous example and reviews the key steps involved. The focus is on summarizing the process of applying the chain rule, which involves taking the derivative of the outer function with respect to the inner function and then multiplying it by the derivative of the inner function with respect to x. The speaker reiterates the use of the power rule in this process and highlights the final result, which is a combination of the derivative of the outer function and the inner function. This paragraph serves as a recap, reinforcing the understanding of the chain rule and its practical use in calculus.

Mindmap
Keywords
💡Chain Rule
The Chain Rule is a fundamental concept in calculus that allows the differentiation of composite functions. It states that the derivative of a composite function is the derivative of the outer function evaluated at the inner function, multiplied by the derivative of the inner function. In the video, the Chain Rule is used to find the derivative of f(g(x)), where f and g are functions defined by the speaker. The application of the Chain Rule is demonstrated through a specific example involving the square root of an expression.
💡Derivative
A derivative in calculus represents the rate of change or the slope of a function at a particular point. It is a foundational concept used to analyze the behavior of functions, such as their increases and decreases, and to find maximum and minimum values. In the video, the derivative is used to determine how one function changes with respect to another, specifically within the context of the Chain Rule.
💡Composition of Functions
The composition of functions is a mathematical operation where one function is applied to the result of another function. It is denoted as f(g(x)), where g(x) is the first function and f is the second function that is applied to the output of g. In the context of the video, understanding the composition of functions is crucial for applying the Chain Rule, as it involves breaking down a complex function into simpler parts to find its derivative.
💡Power Rule
The Power Rule is a basic differentiation rule in calculus that states if a function is of the form x^n, where n is a constant, then its derivative is n*x^(n-1). This rule is used to find the derivative of functions that involve variables raised to a power. In the video, the Power Rule is applied to the inner function g(x) = 3x^2 - x to find its derivative, which is then used in conjunction with the Chain Rule.
💡Square Root
A square root is a mathematical operation that finds a number which, when multiplied by itself, gives the original number. In the context of the video, the square root is used as part of the outer function f(x) = sqrt(x), which is then composed with another function g(x) to find the derivative using the Chain Rule.
💡Function
In mathematics, a function is a relation that pairs each element from one set (the domain) to exactly one element of another set (the codomain). Functions are essential in calculus and are used to model various real-world phenomena. In the video, functions f and g are defined and composed to demonstrate the process of applying the Chain Rule.
💡Differentiation
Differentiation is a process in calculus that finds the derivative of a function, which represents the rate of change or the slope of the function at any given point. It is a fundamental technique used to analyze the behavior of functions. The video focuses on teaching differentiation through the application of the Chain Rule.
💡Inner Function
The inner function in a composition of functions is the function that is being applied first. It is the function that is 'inside' the composition, and its output becomes the input for the outer function. In the video, g(x) is the inner function, which is squared and then subtracted from x to form the composite function.
💡Outer Function
The outer function in a composition of functions is the function that is applied to the result of the inner function. It is the function that 'wraps around' the inner function. In the video, f(x) is the outer function, which takes the result of the inner function g(x) and applies the square root to it.
💡Rate of Change
The rate of change is a fundamental concept in calculus that describes how a quantity changes in relation to another quantity. It is the essence of what a derivative represents, quantifying the instantaneous change or slope of a function at a specific point. The video's focus on differentiation and the Chain Rule is centered around understanding and calculating rates of change for composite functions.
💡Slope
The slope of a function is a measure of how steeply the graph of the function rises or falls. It is closely related to the concept of a derivative, as the derivative of a function at a particular point gives the slope of the tangent line to the graph at that point. The video discusses the derivative, which can be thought of as the slope of the function being examined.
Highlights

The video begins with an introduction to the chain rule formula.

The chain rule is applied in the context of a concrete setting, using a composition of two functions.

The expression f(g(x)) is used to represent a function composed of two other functions, f and g.

The goal is to find the derivative of the composed function with respect to x.

The chain rule states that the derivative of the composed function is the product of the derivative of the outer function with respect to the inner function and the derivative of the inner function with respect to x.

An example is provided to illustrate the application of the chain rule, involving the derivative of the square root of 3x^2 - x.

Functions f and g are defined to represent the composition of f(x) as the square root of x and g(x) as 3x^2 - x.

f(g(x)) is calculated by substituting g(x) into the function f(x).

The derivative of f(x), denoted as f'(x), is found using the power rule and is equal to 1/2 * x^(-1/2).

f'(g(x)) is determined by replacing x with g(x) in the derivative of f(x).

The derivative of g(x), denoted as g'(x), is calculated using the power rule and is equal to 6x - 1.

The final derivative result is 1/2 * g(x)^(-1/2) * (3x^2 - x), which is a combination of the derivatives of the outer and inner functions.

The video emphasizes the importance of understanding the chain rule for solving more complex derivative problems.

The chain rule is a fundamental concept in calculus that allows for the differentiation of composite functions.

The process of applying the chain rule is demonstrated step by step, making it easier for viewers to follow along and understand.

The video uses color-coding to help viewers distinguish between different parts of the mathematical expressions and the process of substitution.

The practical application of the chain rule in solving real-world problems is hinted at, emphasizing its utility beyond abstract mathematics.

Transcripts

Browse More Related Video

The Chain Rule

BusCalc 13 Derivative Chain Rule

Derivative of Inverse Functions | Calculus 1

Chain rule | Derivative rules | AP Calculus AB | Khan Academy

BusCalc 11 Derivative Product Rule

2023 AP Calculus AB FRQ #5

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
DerivativesChain RuleCompositionMathematicsEducationCalculusFunction AnalysisPower RuleTeachingMath Tutorial